There is a dynamic relationship between generalists and specialists. Currently, 4.5% of visits to PCPs result in a referral. A RAND study and my own investigation of the 1998-1999 Community Tracking Survey show that about 10% of individuals are hospitalized at least once each year. How should we model the decision patients face between generalist and specialist care.
Model with perfect information
This is the problem tackled by Blomqvist and Léger in their 2005 paper in the Journal of Health Economics. They have a simple model:
- max_{q,J} U(X,H); J=G or S
- X=I-
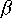 Cj(q)-
Cj(q)-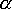
- H=q-
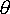
Consumption is represented by ‘X‘, which depends on income ‘I’; the copay rate ![]() , the cost of the procedure Cj(q) and the insurance premium
, the cost of the procedure Cj(q) and the insurance premium ![]() . Health (‘H’) is a function of the amount of medical services chosen (‘q‘) and the underlying health of the person (
. Health (‘H’) is a function of the amount of medical services chosen (‘q‘) and the underlying health of the person (![]() ). This variable is unknown ex ante, but its distribution F(
). This variable is unknown ex ante, but its distribution F(![]() ) is known. The cost of the procedure depends on whether the patient chooses a generalist (j=G) or a specialist (j=S). For each provider type, we have the following first order condition:
) is known. The cost of the procedure depends on whether the patient chooses a generalist (j=G) or a specialist (j=S). For each provider type, we have the following first order condition:
- U_x*(-
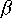 C’j(q)) + U_h=0
C’j(q)) + U_h=0
The authors go on to show that there is a critical value ![]() * above which the individual chooses a specialist and below which the individual chooses a generalist. This critical value will increase when the coinsurance rate (
* above which the individual chooses a specialist and below which the individual chooses a generalist. This critical value will increase when the coinsurance rate (![]() ) increases, since the individual as the individual bears more and more of the cost, they will prefer less dear services. In proposition 3, the authors claim that consumers will choose an inefficiently small
) increases, since the individual as the individual bears more and more of the cost, they will prefer less dear services. In proposition 3, the authors claim that consumers will choose an inefficiently small ![]() * if they are insured. In other words, they will demand too much specialist relative to generalist care. The expected value of the utility function ex ante is:
* if they are insured. In other words, they will demand too much specialist relative to generalist care. The expected value of the utility function ex ante is:
- EU=Int_{
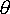 } U[I-
} U[I-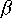 Cj(q(
Cj(q(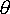 ))-
))-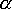 ,q(
,q(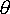 )-
)-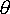 ]dF(
]dF(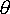 )
)
If the consumer increases the critical value ex post, we see that:
- dE/d
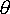 * = V_S(
* = V_S(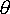 *,
*,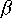 )-V_G(
)-V_G(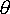 *,
*,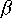 )+(dE/d
)+(dE/d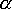 )(d
)(d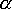 /d
/d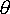 *)
*) - = 0 +(dE/d
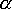 )[C_G(q)-C_s(q)](1-
)[C_G(q)-C_s(q)](1-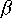 )>0
)>0
Since dE/d![]() <0 (since increasing the insurance premium ceteris paribus will decrease utility) and [C_G(q)-C_s(q)]<0 (since it is assumed that specialist services are more expensive than generalists services, we can show that the equation above is positive as long as the copay rate is less than 100%. This is the mathematical representation of moral hazard.
Model with imperfect information
<0 (since increasing the insurance premium ceteris paribus will decrease utility) and [C_G(q)-C_s(q)]<0 (since it is assumed that specialist services are more expensive than generalists services, we can show that the equation above is positive as long as the copay rate is less than 100%. This is the mathematical representation of moral hazard.
Model with imperfect information
The new model Blomvqist and Léger derive assumes that patients know approximately how sick they are, but only the doctors can know ![]() with certainty. From the paper:
with certainty. From the paper:
“…assume that the distribution F(
) from which illness severity is drawn is bounded by
_o and
_L and is subdivided into L intervals [
_{l-1},
_l] l=1,…L. Although the patient does not observe the exact value of
, we assume that he or she can distinguish between these classes of illnesses; that is, the patient knows in which interval his or her true
is located.”
A doctor must offer an amount of treatment so that for q(![]() _{l-1})>q>q(
_{l-1})>q>q(![]() _{l}). If the doctor does not offer services within this range, the patient will know the doctor is defrauding them by under- or over-providing services. Fee for service doctors still have an incentive to choose the maximum amount of services within the interval, q(
_{l}). If the doctor does not offer services within this range, the patient will know the doctor is defrauding them by under- or over-providing services. Fee for service doctors still have an incentive to choose the maximum amount of services within the interval, q(![]() _{l}), and capitation/salaried doctors still have an incentive to provide the minimum amount of services within the interval, q(
_{l}), and capitation/salaried doctors still have an incentive to provide the minimum amount of services within the interval, q(![]() _{l-1}). The paper continues stating that a managed care contract which specifies different cost sharing parameters
_{l-1}). The paper continues stating that a managed care contract which specifies different cost sharing parameters ![]() _l for each interval will yield a higher expected utility than the optimal conventional contract of the form (
_l for each interval will yield a higher expected utility than the optimal conventional contract of the form (![]() ,
,![]() (
(![]() )).
)).
Analysis
This paper gives very intuitive conclusions and has straight-forward models. Like most health economics models, this one greatly simplifies how medical service provision works. There is only on dimension upon which health can vary and the degree of physician specialization divided into only two discrete categories (i.e.: specialists and generalists). The model claims that appropriately written managed care dominate traditional contracts, but the model does not take into account the cost of information collection needed to correctly establish the copay rate for each of the L subintervals. While this paper will not solve the problems of the medical field, it does put another simple yet insightful model into the healthcare economist’s toolbox.
Blomqvist, Åke; Léger, Pierre Thomas (2005) “Information asymmetry, insurance and the decision to hospitalize” Journal of Health Economics, Vol 24(4), pp. 775-793.